Some Basic Geometric Construction

BASIC GEOMETRIC CONSTRUCTIONS

1. LINE BISECTOR

It is the locus of the points in the plane equidistant from the endpoints of a segment. Therefore it is the locus of all the circumference centres that passes through these endpoints.

The line bisector is a perpendicular line that passes through the midpoint of the segment.

STEPS:
First of all we need to draw a segment. We call it AB.

1. Center your compass in point A, open it further from the middle of the segment AB, and draw an arc.
2. Do the same from point B, where these arcs cross each other we get points 1 and 2.
3. Join 1 and 2, and this way we will get the line bisector of segment AB.
4. 
   

2. PERPENDICULAR LINE TO A LINE FROM A POINT ON IT

Given a line and a point on that line, we will construct a perpendicular line through the given point.
We say that a line is perpendicular to other line when they intersect forming a right angle.

STEPS:
First of all we need to draw a line (r) and mark a point (A) on it.

1. Center your compass in the given point A and draw an arc with the measure you want, where the arc crosses the line we get 1 and 2
2. Get the line bisector between 1 and 2
3. Join 3 and 4, and this way we will get the perpendicular to the given line on point A.
4. 
   

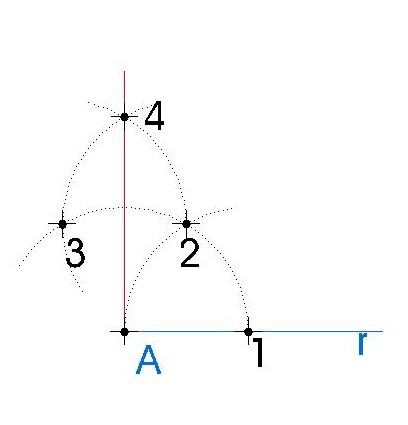
3. PERPENDICULAR LINE TO A LINE FROM AN EXTERNAL POINT

Given a line and a point outside that line, we will construct a perpendicular line through the given point.
We say that a line is perpendicular to other line when they intersect forming a right angle (90º).

STEPS:
First of all we need to draw a line (r) and mark an external point (A). It doesn’t matter where the point is, below or above the line, the steps will be the same.

1. Center your compass in the given point A and draw an arc which crosses the given line r two points called 1 and 2.
2. Get the line bisector between 1 and 2.
3. Join 3 and 4, and this way we will get the perpendicular to the given line on point A.

4. PERPENDICULAR LINE TO A GIVEN RAY ON ITS ENDPOINT

We say that a line is perpendicular to other line when they intersect forming a right angle (90º).

STEPS:
First of all we need to draw a ray (r) and call its endpoint A.

1. Center your compass in the endpoint of the ray (A). Draw an arc with the measure that you want and where this arc crosses the ray we get point 1.
2. Center your compass in point 1 and with the previous measure draw another arc. Where that arc crosses the previous one we get point 2.
3. Center your compass in point 2 and with the same measure draw another arc. Where that arc crosses the first arc we have drawn, we get point 3.
4. Center your compass in point 3 and with the same measure draw another arc. Where that arc crosses the last arc you have drawn, we get point 4.
5. Joining point 4 with the given point A we will get the perpendicular line to the ray on its endpoint.

5. PARALLEL LINE TO A LINE FROM AN EXTERNAL POINT I

We say that a line is parallel to another line when these two lines never cross each other.

STEPS:
First of all we draw a line (r) and draw an external point to it (A)

1. Center your compass in any point of the line (O) and draw an arc that passes through point A.
2. This arc will cross the given line (r) in two points; we will call them P and Q.
3. Draw an arc which radio is the distance between points Q and A taking P as the center. Where that arc crosses the previous one we will get point B.
4. Join point B with the given point A and you will get p, the parallel line to the given line r.

6. PARALLEL LINE TO A LINE FROM AN EXTERNAL POINT II

We say that a line is parallel to another line when these two lines never cross each other.

STEPS:
First of all we draw a line (r) and draw an external point to it (A)

1. Take any two points from the given line (r) and call them P and Q.
2. Draw a circle which radio is the distance between points P and Q taking A as the center.
3. Draw an arc which radio is the distance between points P and A taking Q as the center. Where this arc crosses the circle we will get point B.
4. Join point B with the given point A and you will get p, the parallel line to the given line r

Fuente:http://educacionplasticayvisualeso.wordpress.com/category/tercero-eso-bilingue/page/5/